Question

3. The ratio of the corresponding side lengths of two similar parallelogram

signs is 9:14.
a. What is the ratio of the perimeters? Explain.

Answer 1

The ratio of perimeters is 1 : 1

Explanation:

Given as :

The ratio of corresponding sides length of two parallelogram = 9:14

So, Let The Lengths of the opposite sides of parallelogram = 9 x

And The breadth of the opposite sides of parallelogram = 14 x

Let The perimeter of parallelogram = P

Now, for Parallelogram , its opposite sides are parallel and equal

∴ Perimeter of Parallelogram = 2 × Length + 2 × breadth

or, Perimeter of Parallelogram = 2 × 9 x + 2 × 14 x

or, Perimeter of Parallelogram = 18 x + 28 x

or, Perimeter of Parallelogram = 46 x

Now, Since both the parallelogram have same side length ratio , so

The perimeter of both is same = 46 x

So ,
`(\textrm perimeter of parallelogram 1)/(\textrm perimeter of parallelogram 2)` =
`(46 x)/(46 x)`

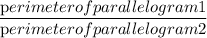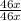
Or,
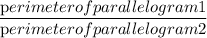`(\textrm perimeter of parallelogram 1)/(\textrm perimeter of parallelogram 2)` =1 : 1

Hence The ratio of perimeters = 1 : 1 Answer