Question

For what period of time during the dive is the diver's center of mass higher than the diving board, which is 6 feet above the water? MODULE 4.4 ALGEBRA 2 DIVING Relative to the surface of the water, the height h in feet of a diver's center of mass during a dive is modeled by the function h(t) = -16t + 14t + 10 where t is the time in seconds since the dive began

Answer 1

For around 1.1018 seconds, the diver's center of mass higher than the diving board, which is 6 feet above the water.

Explanation:

We are given the following information in the question:

Relative to the surface of the water, the height h in feet of a diver's center of mass during a dive is modeled by the function h(t).

`h(t) = -16t^2 + 14t + 10`

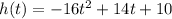
where h is in feet and t is the time in seconds since the dive began.

Since this is relative to water level, we treat water level as 0.

We have to find the period of time during the dive is the diver's center of mass higher than the diving board, which is 6 feet above the water.

Thus, h(t) = 6 feet

`h(t) = 6 =-16t^2 + 14t + 10\\-16t^2 + 14t + 4 = 0`

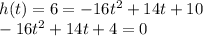
Using the quadratic formula, to solve this quadratic equation:

`at^2 + bt + c = 0\\\\t = \displaystyle(-b\pm √(b^2-4ac))/(2a)\\\\\text{Putting }a = -16, b = 14, c = 4\\\\t =  \displaystyle(-14\pm √((14)^2-4(-16)(4)))/(2(-16))\\\\t = -0.2268, 1.1018`

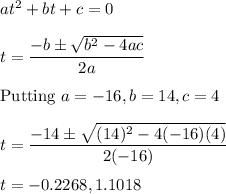
Thus, for around 1.1018 seconds, the diver's center of mass higher than the diving board, which is 6 feet above the water.