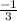Question

Show all work to solve 3x^2 − 5x − 2 = 0

Answer 1

x=2

Explanation:

Step 1: Factor left side of equation.

(3x+1)(x−2)=0

Step 2: Set factors equal to 0.

3x+1=0 or x−2=0

Answer 2

The solution of quadratic equation
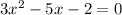`3 x^(2)-5 x-2=0` is
`x=2 \text { or } (-1)/(3)`

Solution:

Given, equation is
`3 x^(2)-5 x-2=0`

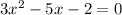
We have to solve the above given quadratic equation.

Now, take the given quadratic equation

`\rightarrow 3 x^(2)-5 x-2=0`

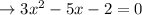
Splitting “-5x” as -6x + x

`\rightarrow 3 x^(2)-6 x+x-2=0`

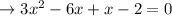
Take “3x” as common term from first two terms

`\rightarrow 3 x(x-2)+1(x-2)=0`

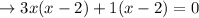
Take (x - 2) as common

`\rightarrow(x-2)(3 x+1)=0`

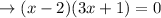
Equating to zero we get,

`\begin{array}{l}{\rightarrow x-2=0 \text { or } 3 x+1=0} \\\\ {\rightarrow x=2 \text { or } 3 x=-1} \\\\ {\rightarrow x=2 \text { or } x=(-1)/(3)} \\\\ {\rightarrow x=2 \text { or } (-1)/(3)}\end{array}`

Hence, the roots the quadratic equation are 2 and
`(-1)/(3)`