Answer:
h=60 and v=54000 cm³.
Explanation:
Let h be the height of the box kite,
Assume that the length of one face of base is 30 cm and another face of the base is 30 cm.
The total area of the kite box that is covered by the fabric
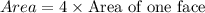
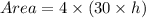
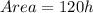
The area of 4 sides of the kite is 7200.
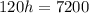
Divide both sides by 120.
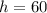
The height of the box is 60 cm.
The volume of a rectangular prism.
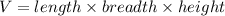

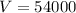
Therefore, the height of the kite is 60 units and volume of the volume inside the kite is 54000 cm³.