Question

Suppose f '' is continuous on (−[infinity], [infinity])

(1) If f '(-1) = 0 and f ''(-1) = -7, what can you say about f?

a)At x = -1, f has local maximum.

b)At x = -1, f has a local minimum.

c) At x = -1, f has not a maximum or minimum.

d) There is not enough information.

(2) If f '(4) = 0 and f ''(4) = 0, what can you say about f?

a) At x = 4, f has local maximum.

b) At x = 4, f has a local minimum.

c) At x = 4, f has not a maximum or minimum.

d) There is not enough information.

Answer 1

c

Explanation:

Answer 2

Answer with Step-by-step explanation:

We are given that a function f(x) is continuous on (
`-\infty,\infty`).

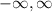1.f'(-1)=0 and f''(-1)=-7

We have to find information about f.

When f'(-1)=0 and f''(-1)=-7 < 0

Then, function is maximum at x=-1.

Therefore, at x=-1, f has local maximum.

Answer:a)at x=-1 ,f has local maximum.

2.) if f'(4)=0 and f''(4)=0

We know that when f''(x)=0 then test fails then the function has not maximum or minimum.

Therefore, at x=4 , f has not a maximum or minimum.

Answer:c) at x=4, f has not a maximum or minimum.