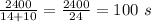Answer:
Time spent rowing down stream
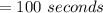
Speed of boat in still water
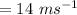
Explanation:
Let speed of boat in still water be
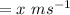
Speed of current

Speed of boat down stream =
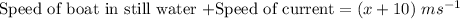
Distance rowed down stream = 2400 m
Time spent rowing down stream =
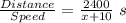 =
=
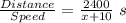
Speed of boat up stream =
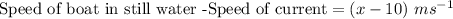
Distance rowed up stream =
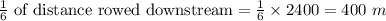
Time spent rowing up stream =

We know that,

So,
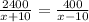
Cross multiplying

Dividing both sides by

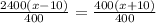
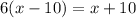
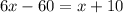
Adding 60 to both sides.

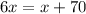
Subtracting both sides by

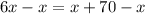
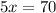
Dividing both sides by 5.

∴
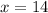
Speed of boat in still water

Time spent rowing down stream =