Question

1. Is there sufficient evidence in the data to conclude that the average time to deliver a pizza, once the order is placed, is greater than 25 minutes? Perform an appropriate statistical analysis and attach any supporting output from JMP.

Answer 1

pizza hut

Explanation:

when I order pizza hut it will say 25 min delivery but usually takes 35, therefore the pizza does not come on time ever. As a ex employee of a local pizza shop I can also confirm to you that the drivers do not care about your food

Answer 2

There's no enough evidence to conclude that the average time is not greater than 25 minues.

The complete question is

"A pizza restaurant manager claims that the average home delivery time for their pizza is no more than 25 minutes. A random sample of 49 home delivery pizzas was collected. The sample mean was found to be 26.5 minutes and the standard deviation was found to be 4 minutes. Is there evidence to reject the manager's claim at alpha =.05?"

First, we have to define the system of hypothesis.

`H_(0): \mu =25\\ H_(a): \mu >25`

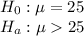
(Remeber that this system is formed by a null hypothesis and an alternative hypothesis)

Then, we find the z-value:

`Z=(26.5-25)/(4)=(1.5)/(4) =0.375`

Now, you need to use a table to find the p-value (for a 0.05 level of confidence), which is the probability value.

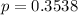`p=0.3538`, this is the value assigned for the z-value we found.

Noitce that the p-value isn't greater than the z-value, that means we don't have enough evidence to reject the null hypothesis.