Answer:
0.36 rad/s
Step-by-step explanation:
from the question we are given the following:
width of the door (w) = 1 m
length of the door (L) = 2 m
mass of the door (Md) = 40 kg
mass of mud (Mm) = 0.7 kg
velocity of the mud (V) = 14 m/s
angular speed = ?
we can get the angular speed by equating the initial angular momentum to the final angular momentum
initial angular momentum of the mud = final angular momentum of the mud and door
m x v x \frac{r}{2} = (
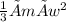 + m
+ m
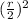 ) x ω
) x ω
note that r is divided by 2 because the mud hit the door at its center which is half of its width
0.7 x 14 x \frac{1}{2} = (
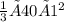 + (0.7 x
+ (0.7 x
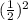 )) x ω
)) x ω
ω =
/(3) × 40 × 1 ^(2)]() + (0.7 x
+ (0.7 x
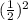 ))}[/tex]
))}[/tex]
= 0.36 rad/s