Question

A driver traveling in her 16-foot SUV at the speed limit of 30 mph was arrested for running a red light at 15th and Main, an intersection that is 60 feet wide. The driver claimed innocence, on the grounds that the traffic signals were not set properly. The yellow light was on for the standard 4 seconds. The SUV driver's reaction time is assumed to be 1.5 seconds. Comfortable deceleration is at a rate of 10 feet/second2. Did a dilemma zone exist on this intersection approach? If so, how long was it? Assume the vehicle must completely clear the intersection to avoid "running a red light." Yes, D 63 ft. 7.

Answer 1

(a) Yes

(b) 102.8 ft

Step-by-step explanation:

(a)First let convert mile per hour to feet per second

30 mph = 30 * 5280 / 3600 = 44 ft/s

The time it takes for this driver to decelerate comfortably to 0 speed is

t = v / a = 44 / 10 = 4.4 (s)

given that it also takes 1.5 seconds for the driver reaction, the total time she would need is 5.9 seconds. Therefore, if the yellow light was on for 4 seconds, that's not enough time and the dilemma zone would exist.

(b) At this rate the distance covered by the driver is

`s = v_0t + (at^2)/(2)`

`s =44*1.5 + 44(4.4) - (10*4.4^2)/(2) = 162.8 (ft)`

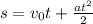
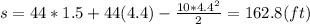
Since the intersection is only 60 feet wide, the dilemma zone must be

162.8 - 60 = 102.8 ft