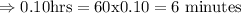Question

Alex cycles at a speed of 10 miles per hour He cycles 6 miles from home to

school. If he increases his speed by 2 miles per hour, how much Taster will he
reach his school?​

Answer 1

If Alex increases his speed by 2 miles per hour from 10 miles per hour he will be 0.01 hrs or 6 minutes faster that is will take 6 minutes less to reach school.

Solution:

Given that

Alex cycles at a speed of 10 miles per hour; Alex cycles 6 miles from home to school.

Need to calculate how much faster will Alex reach to school if he increase its speed by 2 miles per hour . Formula for time is as follows ,

`\text { Time }=\frac{\text { Distance covered }}{\text { speed }}`

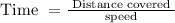
Lets calculate time taken by Alex to reach school at normal speed that is 10 miles per our

As distance covered from home to school is 6 miles

Distance covered = 6 miles

Normal Speed = 10 miles per hour

Substituting the given values in formula of time we get

Time taken at normal speed
`\mathrm{T}_{\mathrm{N}}=(6)/(10)=0.6 \text { hours }`

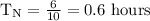
Now calculate time taken by Alex to reach school when speed is increased by two

Distance covered = 6 miles

Increase Speed =
`10 + 2 = 12 \text { miles }`

Substituting the given values in formula of time we get

Time taken at Increased speed
`\mathrm{T}_{\mathrm{I}}=(6)/(10)=0.5 \text { hours }`

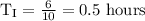
Difference in time taken =
`\mathrm{T}_{\mathrm{N}}-\mathrm{T}_{\mathrm{I}}=0.6-0.5=0.10 \mathrm{ hrs }`

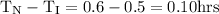
As 1 hour = 60 minutes

`\Rightarrow 0.10 \mathrm{hrs}=60 \mathrm{x} 0.10=6 \text { minutes }`