Question

To view an interactive solution to a problem that is similar to this one, select Interactive Solution 7.24. A 0.0146-kg bullet is fired straight up at a falling wooden block that has a mass of 2.55 kg. The bullet has a speed of 816 m/s when it strikes the block. The block originally was dropped from rest from the top of a building and had been falling for a time t when the collision with the bullet occurs. As a result of the collision, the block (with the bullet in it) reverses direction, rises, and comes to a momentary halt at the top of the building. Find the time t. Number Units.

Answer 1

0.25223 seconds.

Step-by-step explanation:

`m_1` = Mass of bullet = 0.0146 kg

`m_2` = Mass of block = 2.55 kg

v = Combined velocity

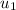`u_1` = Velocity of bullet = 816 m/s

g = Acceleration due to gravity = 9.81 m/s²

As linear momentum is conserved

`m_1u_1 + m_2u_2 =(m_1 + m_2)v`

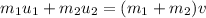
Now
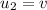`u_2=v` as the block (with the bullet in it) reverses direction and rises,

`m_1u_1 + m_2v =(m_1 + m_2)v\\\Rightarrow m_1u_1=(m_1 + m_2)v-m_2v\\\Rightarrow 0.0146* 816=(0.0146 + 2.55)v-(-2.55v)\\\Rightarrow 11.9136=2.2646v+2.55v\\\Rightarrow 11.9136=4.8146v\\\Rightarrow v=(11.9136)/(4.8146)\\\Rightarrow v=2.47447\ m/s`

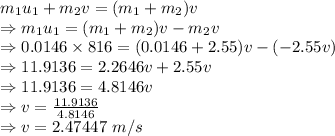
Equation of motion

`v=u+at\\\Rightarrow t=(v-u)/(a)\\\Rightarrow t=(2.47447-0)/(9.81)\\\Rightarrow t=0.25223\ s`

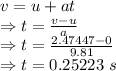
The time t is 0.25223 seconds.