Question

A manufacturing company uses an acceptance scheme on items from a production line before they are shipped. The plan is a two-stage one. Boxes of 25 items are readied for shipment, and a sample of 3 items is tested for defectives. If any defectives are found, the entire box is sent back for 100% screening. If no defec- tives are found, the box is shipped. (a) What is the probability that a box containing 3 defectives will be shipped? (b) What is the probability that a box containing only 1 defective will be sent back for screening?

Answer 1

The probability that a box containing 3 defectives will be shipped is 0.6696.

The probability that a box containing only 1 defective will be sent back for screening is 0.12

Explanation:

Consider the provided information.

Boxes of 25 items are readied for shipment, and a sample of 3 items is tested for defectives.

Part (A) What is the probability that a box containing 3 defectives will be shipped?

Let x represents the number of defective item among the 3 item.

According to hyper geometric distribution:

`h(x; N, n, k) = \frac{\binom{k}{x} \binom{N-k}{n-x}}{\binom{N}{n}}`

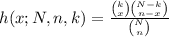
Where n is randomly selected without replacement from a population of N items. k items can be classified as successes, and N - k items can be classified as failures.

Therefore, N = 25, n = 3 and k=3 in above formula.

The probability that box deliver 3 defective item.

P(No defective item among selected 3)=P(x=0)

`P(x=0) = \frac{\binom{3}{0} \binom{25-3}{3-0}}{\binom{25}{3}}`

`P(x=0) =0.6696`

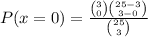
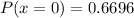
Therefore, the probability that a box containing 3 defectives will be shipped is 0.6696.

Part (B) What is the probability that a box containing only 1 defective will be sent back for screening?

Substitute, N = 25, n = 3 and k=1 in above formula.

The probability that a box containing only 1 defective will be sent back for screening

`P(x=1) = \frac{\binom{1}{1} \binom{25-1}{3-1}}{\binom{25}{3}}`

`P(x=1) =0.12`

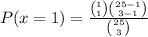
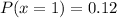
Hence, the probability that a box containing only 1 defective will be sent back for screening is 0.12