Answer:
Option 3.
Explanation:
It is given that a triangle sits on a line and forms 2 exterior angles on the left and right of the triangle of (2h) degrees.
The top interior angle of the triangle is 40 degrees.
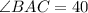
From the given figure it is clear that
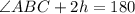 (Supplementary angle)
(Supplementary angle)
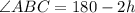
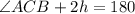 (Supplementary angle)
(Supplementary angle)
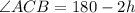
According to the angle sum property of triangle, the sum of all interior angles of a triangle is 180 degree.
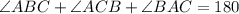

Combine like terms.
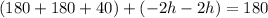
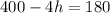
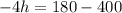

Divide both sides by -4.
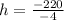

The value of h is 55.
Therefore, the correct option is 3.