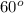2 Answers

Benja Garrido · Answer 1 · 2020-07-04T09:03:59+0000

Answer:

<c =60

Explanation:

The angles of a triangle need to add up to 180 degrees. if you subtract 40 and 80 from 180 you will come up with the answer 60

Michael Zhang · Answer 2 · 2020-07-08T04:25:44+0000

Answer : The correct option is, (B)

Step-by-step explanation :

As we are given a triangle ABC.

Given:

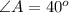
$\angle A=40^o$

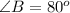
$\angle B=80^o$

Property of triangle : The sum of the interior angle of a triangle is equal to
180^o

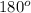
That means,

$\angle A+\angle B+\angle C=180^o$

Now put all the value of angle in this expression, we get:

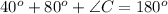
$40^o+80^o+\angle C=180^o$

$120^o+\angle C=180^o$

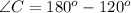
$\angle C=180^o-120^o$

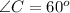
$\angle C=60^o$

Therefore, the value of
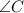$\angle C$ is,

Please log in or register to add a comment.