Question

Below is a sequence of numbers:

0.5, 3, 5.5, 8, …
Calculate:
(a) the 8th term,
Answer: ______________
(b) the 100th term,
Answer: ______________
(c) which term has a value of 148.

Answer 1

a) 18

b)248

c) 58

Explanation:

The formula is:

`a_(n)` =
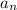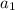`a_(1)` + (n-1)d

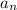`a_(n)` Is the number at the n term

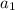`a_(1)` Is the first number in the sequence

n Is the number for the term

d is the common difference. How much is added each time in the sequence. In this case, 2.5

Put in the numbers that you know and solve for the unknown.

A:

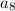`a_(8)` = 0.5 + (8 -1)(2.5)

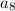`a_(8)` = 0.5 +(7)(2.5)

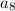`a_(8)` = 0.5 + 17.5

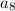`a_(8)` = 18

B:

We follow the same pattern, but we are now looking for
`a_(100)`.

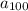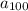`a_(100)` = 0.5 + (100-1)(2.5)

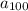`a_(100)` = 0.5 + 247.5

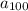`a_(100)` = 248

C:

This time we know the value of the number in the sequence, we do not know which term it is.

`a_(n)` =
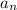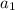`a_(1)` + (n - 1)d Put in what we know

148 = 0.5 + (n -1)2.5 Distribute the 2.5

148 = 0.5 + 2.5n - 2.5 Combine like terms

148 = 3 + 2.5n Subtract 3 from both sides of the equation

145 = 2.5n Divide both sides by 2.5

58 = n

Answer 2

for part c im not really sure if the answer is 59 or 60