Question

The physical plant at the main campus of a large state university recieves daily requests to replace florecent lightbulbs. The distribution of the number of daily requests is bell-shaped and has a mean of 60 and a standard deviation of 11. Using the Empirical Rule rule, what is the approximate percentage of lightbulb replacement requests numbering between 60 and 71?

Answer 1

Answer: 34%

Explanation:

According to the Empirical rule,

About 68% of the population lies with in one standard deviation from the mean.

i.e. About 34% of the population lies above one standard deviation from the mean .

and About 34% of the population lies below one standard deviation from the mean.

Given : The distribution of the number of daily requests is bell-shaped ( i.e. Normally distribution) and has a mean of 60 and a standard deviation of 11.

i.e.
`\mu=60\ \ \sigma=11`

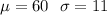
Using the Empirical Rule rule, 34% of the population of lightbulb replacement requests lies above one standard deviation from the mean .

i.e. About 34% of the population of lightbulb replacement requests lies between
`\mu` and
`\mu+\sigma`

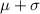
i.e. About 34% of the population of lightbulb replacement requests lies between
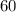`60` and
`60+11`

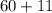
i.e. About 34% of the population of lightbulb replacement requests lies between 60 and 71

Hence, the approximate percentage of lightbulb replacement requests numbering between 60 and 71 = 34%