Question

Which number is rational? −49√ −27√ −3√ -12√

Answer 1

−49√

Step-by-step explanation:

Answer 2

The number -12√ is rational.

Step-by-step explanation:

A rational number is any number that can be expressed as the quotient or fraction
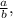`\( (a)/(b) \),` where ( a ) and ( b ) are integers and ( b ) is not equal to zero. In the provided options, -12√ can be simplified to a rational number. To show this, we can express -12√ as
`\(-(12√(1))/(√(1))\),` which simplifies to
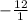`\(-(12)/(1)\)` or simply -12. Therefore, -12√ is rational.

Understanding the nature of numbers is crucial in mathematics. In this case, recognizing that the square root of 1 is 1, we can simplify the expression and identify the rational number. This concept is foundational in various mathematical fields, particularly in algebra and number theory.

It's worth noting that the other options, -49√, -27√, and -3√, involve the square roots of numbers that are not perfect squares, and therefore, these expressions cannot be simplified to rational numbers. The square root of a non-perfect square is an irrational number, meaning it cannot be expressed as a simple fraction of two integers.