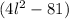Question

Please Help

Find the product of each sum and difference. (2l + 9)(2l – 9)

(a) 4l2 + 18l – 81

(b) 4l2 – 81

(c) 4l2 + 81

(d) 4l

Answer 1

Option c
`4l^2-81`

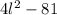
Step-by-step explanation:

As we have given in the question

`Sum=(2l+9)*(2l-9)`

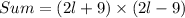
So by using the formula

`(a-b) (a+b) = a^2+ab-ba-b^2`

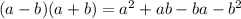
So the equation will become

`(a+b)(a-b)=a^2-b^2`

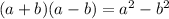
So according to question, we have ,

a = 2l and b = 9

So,

`(2l+9)(2l-9)=(4l^2-81)`

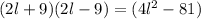
And required Sum=
`(4l^2-81)`

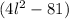
So the final answer of the question which is required is Sum=
`(4l^2-81)`

Answer 2

Answer:(b). 4I2-81

Step-by-step explanation:(2I+9)(2I-9)

=4I2-18I+18I-81

=4I2-81