Question

Does there exist a pair of consecutive integers whose reciprocals sum to 3/4? Explain how you know.

Answer 1

NO

Explanation:

Let the consecutive numbers be x and x + 1

Given condition is their reciprocals sum is equal to 3/4

`(1)/(x) +(1)/(x+1) =(3)/(4)`

`(2x+1)/(x^2+x)=(3)/(4)`

`8x+4=3x^2+3x`

`3x^2-5x-4=0`

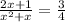
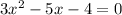
Find the discriminant for the above quadratic equation

Foe a given quadratic equation
`ax^2+bx+c=0` the discriminant =
`b^2-4ac`

Here a = 3,b = -5,c = -4

Discriminant =
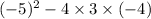`(-5)^2-4* 3* (-4)` =78 >0

If a quadratic equation has discriminant > 0 it has distinct real roots

The roots are
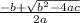`(-b+√(b^2-4ac) )/(2a)` and
`(-b-√(b^2-4ac) )/(2a)`

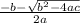
Here the roots are
`(5+√(78) )/(6) and (5-√(78) )/(6)`

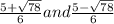
They are not integers So there are no consecutive pair of integers which follow the given condition