Question

The random variable X is exponentially distributed, where X represents the time it takes for a person to choose a birthday gift. If X has an average value of 24 minutes, what is the probability that X is less than 32 minutes? (Do not round until the final step. Round your answer to 3 decimal places.)

Answer 1

The probability that X is less than 32 minutes is 0.736.

Explanation:

Given : The random variable X is exponentially distributed, where X represents the time it takes for a person to choose a birthday gift. If X has an average value of 24 minutes.

To find : What is the probability that X is less than 32 minutes?

Solution :

If X has an average value of 24 minutes.

i.e.
`\lambda=24`

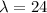
The random variable X is exponentially distributed, where X represents the time it takes for a person to choose a birthday gift.

The exponentially function is
`(1)/(\lambda)e^{-(x)/(\lambda)}`

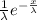
The function form according to question is

`f(x)=\{(1)/(24)e^{-(x)/(24)}, x>0\}`

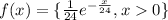
The probability that X is less than 32 minutes is

`P[x<32]=1-e^{-(32)/(24)}`

`P[x<32]=1-0.26359`

`P[x<32]=0.736`

Therefore, the probability that X is less than 32 minutes is 0.736.