Answer:
C.
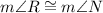 ; this can be confirmed by translating point R to point N
; this can be confirmed by translating point R to point N
Step-by step explanation:
We are given that
Triangle LMN in which
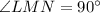
Triangle PQR is a dilation of triangle LMN by scale factor of 2 from the center of dilation at point M.
We have to find the statement that can be used to prove that triangle LMN is similar to triangle PQR by AA similarity postulates.
Dilation: It is that transformation in which shape of figure does not change but the size of figure changes.
Initial figure and final figure are similar in dilation .
Measure of angles or sides does not change only size changes in dilation transformation.
AA similarity postulate: When two angles of triangle are congruent to its corresponding angles of other triangle then, two triangle are similar by AA similarity postulate.

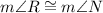
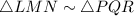
Reason: AA similarity postulate
Hence, option C is true.