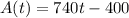Answer: The equation for the relationship between the altitude and number of hours is
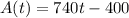
Explanation:
Since we have given that
Speed = 740 m/hour
Number of hours = 1.5 hours
Let t be the number of hours.
A be the altitude relative to sea level.
So, According to question, it becomes,
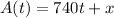
where, x is the initial height of Noa.
After 1.5 hours, altitude becomes 710 meters.

Hence, the equation for the relationship between the altitude and number of hours is