Question

Henry is asked to find the exact value of cos 10pi/3. His steps are shown below.

1. Subtract 2pi from
10pi/3 as many times as possible:
10pi/3 - 2pi = 4pi/3
2. Find the reference angle for 4pi/3 : 3pi/2 - 4pi/3 = pi/6
3. The cosine value for pi/6 is radical 3/2
4. The cosine value is positive because pi/6 is in the first quadrant.

Which of the following describes Henry's errors?
(following shown in picture)

Answer 1

First option

The reference angle should be pi/3, and the sign of the value should be negative.

I hope this helped you

Answer 2

A) The reference angle should be
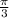`(\pi )/(3)`, and the sign of the value should be negative.

Explanation:

cos(
`(10\pi )/(3)`)

Remove full rotations of 2π until the angle is between 0 and 2
`\pi`.

cos(
`(4\pi )/(3)`)

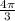Apply the reference angle by finding the angle with equivalent trig values in the first quadrant. Make the expression negative because cosine is negative in the third quadrant.

-cos(
`(\pi )/(3)`)

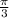The exact value of cos(
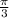`(\pi )/(3)`) is
`(1)/(2)`.

−
`(1)/(2)`