Question

Find a quadratic function with roots x = 8 and x = 9.

Answer 1

y=x^2-17x+72

Explanation:

You have to Work backwards to find the factors from the roots, then multiply the factors together

Answer 2

The quadratic function with roots x = 8 and x = 9 is given as
`x^(2)-17 x+72=0`

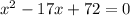
Solution:

Given that the roots of function are x = 8 and x = 9

We need to find the quadratic function

Now, we know that, quadratic equation is given by
`x^(2)-(a+b) x+a b=0`

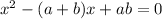
where a and b are roots of that quadratic equation.

Here a = 8 and b = 9

By substituting the "a" and "b" values in general quadratic function we get,

`\begin{array}{l}{x^(2)-(8+9) x+8 * 9=0} \\\\ {x^(2)-(17) x+72=0} \\\\ {x^(2)-17 x+72=0}\end{array}`

Hence the required quadratic function is found out