Answer:
The cosine of angle PRQ is 0.50043
Explanation:
From figure :
In triangle PRQ
angle RPQ = 90° - 60° = 30°
Angle PQR = 90°
SO, angle PRQ = 180° - ( 90° + 30° )
Or, angle PRQ = 60°
Now Cos ∠ PRQ =
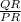
I.e Cos 60° =
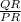
Now cos ∠ RPQ =
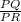
Or, Cos 30° =
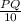
So, PQ = 10 × Cos 30°
Or, PQ = 8.66
Now QR =
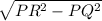
Or, QR =

So , QR = 5.00043
∵, Cos ∠ PRQ =
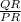
∴ Cos ∠ PRQ =

I,e Cos ∠ PRQ = 0.50043
Hence The cosine of angle PRQ is 0.50043 Answer