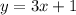For this case we have that by definition, the equation of a line in the slope-intersection form is given by:
Where:
m: Is the slope
b: Is the cut-off point with the y axis
According to the data of the statement we have two points through which the line passes:
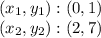
We found the slope:

Thus, the equation is of the form:
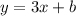
We substitute one of the points and find "b":
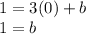
Finally, the equation is:
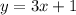
Answer: