To solve this problem we must keep in mind the concepts related to angular kinematic equations. For which the angular velocity is defined as
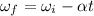
Where
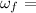 Final angular velocity
Final angular velocity
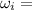 Initial angular velocity
Initial angular velocity
 Angular acceleration
Angular acceleration
t= time
In this case we do not have a final angular velocity, then
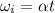
Re-arrange for


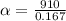
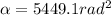
Therefore the mangitude of the angular aceleration is 5449.1rad/s²