Answer:
The solution set is { 66.6°, 113.4°, 192.6°, 347.4° }.
Explanation:
Given:
The given equation in the interval (0°,360°) is given as:

Adding -2 both sides, we get
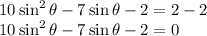
This is a quadratic equation in
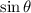 of the form
of the form
 . Solve it using the quadratic formula given as:
. Solve it using the quadratic formula given as:
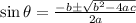
Here,
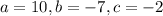
Therefore,
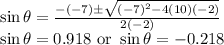
Now, taking inverse of the sine values, we get
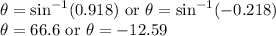
But, we need to find
 in the interval (0°,360°).
in the interval (0°,360°).

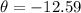 is in the fourth quadrant, So, its positive value is:
is in the fourth quadrant, So, its positive value is:
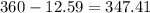 °
°
Also, from unit circle, sine of the angle in third and fourth quadrants are same. So,
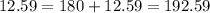 °
°
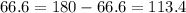 °
°
Therefore, the set of angles for which the above equation is satisfied in the interval (0°,360°) is { 66.6°, 113.4°, 192.6°, 347.4° }.