Question

The graph below represents which system of inequalities? (2 points)

graph of two intersecting lines. One line is solid, and goes through the points 0, negative 2 and 1, 0 and is shaded in below the line. The other line is dashed, and goes through the points 0, 3, 3, 0 and is shaded in above the line.

Group of answer choices

y > 2x − 3
y > −x − 3

y < 2x − 2

y < −x + 3


y ≤ 2x − 2
y > −x + 3

None of the above

Answer 1

Solid...(-3,0)(-4,-1)...shaded below the line

slope = (-1-0) / (-4-(-3) = -1/(-4 + 3) = -1/-1 = 1

y = mx + b

-1 = 1(-4) + b

-1 = -4 + b

-1 + 4 = b

3 = b

this line is : y < = x + 3 <== (thats less then or equal)

====================

solid...(1,1)(2,-1)...shaded below the line

slope = (-1-1) / (2-1) = -2/1 = -2

y = mx + b

1 = -2(1) + b

1 = -2 + b

1 + 2 = b

3 = b

this line is : y < = -2x + 3 <== (thats less then or equal)

Explanation:

Answer 2

Option C, y ≤ 2x − 2 and y > −x + 3

Explanation:

If a line passes through two points, then the equation of line is

`y-y_1=(y_2-y_1)/(x_2-x_1)(x-x_1)`

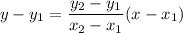
One line is solid, and goes through the points (0, -2) and (1, 0) and is shaded in below the line. The equation of related line is

`y-(-2)=(0-(-2))/(1-0)(x-0)`

`y+2=2x`

`y=2x-2`

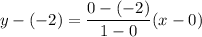
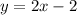
Everything is to the below of the line is shaded and the related line is solid. So, the sign of inequality must be ≤.

`y\le 2x-2`

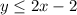
The other line is dashed, and goes through the points (0, 3), (3, 0) and is shaded in above the line. The equation of related line is

`y-3=(0-3)/(3-0)(x-0)`

`y-3=-x`

`y=-x+3`

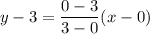
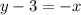
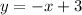
Everything is to the above of the line is shaded and the related line is dashed. So, the sign of inequality must be >.

`y>-x+3`

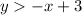
The system of inequity is

y ≤ 2x − 2

y > −x + 3

Therefore, correct option is C.