Answer: D.
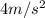
Step-by-step explanation:
The question is incomplete, please remember to write the whole question. However, the the complete question is as follows:
Mars has a mass 1/10 that of Earth and a diameter 1/2 that of Earth. The acceleration of a falling body body near the surface of Mars is most nearly:
A.
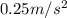
B.
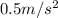
C.

D.
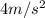
E.
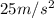
Let's begin by the fact that on Earth, the gravity force
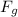 that acts on a falling object is given by:
that acts on a falling object is given by:
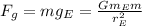 (1)
(1)
Where:
 is the mass of the falling body
is the mass of the falling body
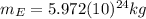 is the mass of the Earth
is the mass of the Earth
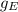 is the acceleration due gravity on Earth
is the acceleration due gravity on Earth
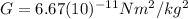 is the Universal Gravitational constant
is the Universal Gravitational constant
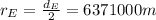 is the Earth's radius and
is the Earth's radius and
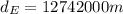 is its diameter
is its diameter
Simplifying (1) we have:
 (2)
(2)
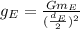 (3)
(3)
Now, in the case of Mars we have:
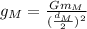 (4)
(4)
Where:
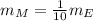
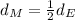
Substituting these on (4):
 (5)
(5)
Simplifying:
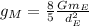 (6)
(6)
Then:
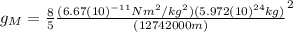 (7)
(7)
Finally:
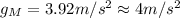 This is most nearly the acceleration of a falling body near the surface of Mars
This is most nearly the acceleration of a falling body near the surface of Mars