Question

Two ice skaters, each with a mass of 72.0 kg, are skating at 5.45 m/s when they collide and stick together. If the angle between their initial directions was 105°, determine the components of their combined velocity (in m/s) after the collision. (Let the initial motion of skater 1 be in the positive x-direction and the initial motion of skater 2 be at an angle of 105°measured counterclockwise from the positive x-axis.) vx, f = ___________ m/s vy, f = ___________ m/s

Answer 1

The skater 1 and skater 2 have a final speed of 2.02m/s and 2.63m/s respectively.

Step-by-step explanation:

To solve the problem it is necessary to go back to the theory of conservation of momentum, specifically in relation to the collision of bodies. In this case both have different addresses, consideration that will be understood later.

By definition it is known that the conservation of the moment is given by:

`m_1v_1+m_2v_2=(m_1+m_2)v_f`

Our values are given by,

`m_1=m_2=72Kg`

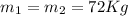
As the skater 1 run in x direction, there is not component in Y direction. Then,

Skate 1:

`v_(x1)=5.45m/s`

`v_(y1)=0`

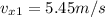
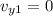
Skate 2:

`v_(x2) = 5.45*cos105= -1.41m/s`

`v_(y2) = 5.45*sin105 = 5.26m/s`

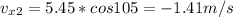
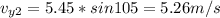
Then, if we applying the formula in X direction:

m_1v_{x1}+m_2v_{x2}=(m_1+m_2)v_{fx}

75*5.45-75*1.41=(75+75)v_{fx}

Re-arrange and solving for v_{fx}

v_{fx}=\frac{4.04}{2}

v_{fx}=2.02m/s

Now applying the formula in Y direction:

`m_1v_(y1)+m_2v_(y2)=(m_1+m_2)v_(fy)`

`0+75*5.25=(75+75)v_(fy)`

`v_(fy)=(5.25)/(2)`

`v_(fy)=2.63m/s`

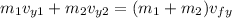
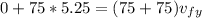
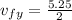
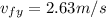
Therefore the skater 1 and skater 2 have a final speed of 2.02m/s and 2.63m/s respectively.