Question

The average rate of change of the function g(x)=3÷2x+1 over the interval -2<x<8​

Answer 1

The rate of change is -1.5.

Explanation:

Given : Function
`g(x)=(3)/(2)x+1`

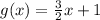
To find : The average rate of change of the function over the interval -2<x<8​?

Solution :

The average rate of function f(x) between the interval a and b is given by,

`\text{Rate of change}=(f(b)-f(a))/(b-a)`

Here,
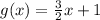`g(x)=(3)/(2)x+1` and a=-2 and b=8

`\text{Rate of change}=(g(-2)-g(8))/(8-(-2))`

`g(-2)=(3)/(2)(-2)+1`

`g(-2)=-3+1`

`g(-2)=-2`

`g(8)=(3)/(2)(8)+1`

`g(8)=12+1`

`g(8)=13`

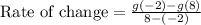
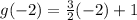
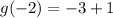
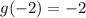
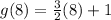
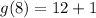
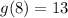
Substitute,

`\text{Rate of change}=(-2-(13))/(8-(-2))`

`\text{Rate of change}=(-15)/(10)`

`\text{Rate of change}=-1.5`

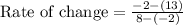
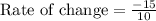
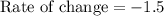
Therefore, the rate of change is -1.5.