Question

A 8.34 × 103-kg lunar landing craft is about to touch down on the surface of the moon, where the acceleration due to gravity is 1.60 m/s2. At an altitude of 162 m the craft's downward velocity is 18.2 m/s. To slow down the craft, a retrorocket is firing to provide an upward thrust. Assuming the descent is vertical, find the magnitude of the thrust needed to reduce the velocity to zero at the instant when the craft touches the lunar surface.

Answer 1

21870.3156 N

Step-by-step explanation:

u = Initial velocity

v = Final velocity

s = Displacement

a = Acceleration

g = Acceleration due to gravity = 1.6 m/s²

Equation of motion

`v^2-u^2=2as\\\Rightarrow a=(v^2-u^2)/(2s)\\\Rightarrow a=(0^2-18.2^2)/(2* 162)\\\Rightarrow a=-1.02234\ m/s^2`

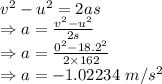
The acceleration of the craft should be 1.02234 m/s²

`F=ma\\\Rightarrow F=8.34* 10^3* 1.02234\\\Rightarrow F=8526.3156\ N`

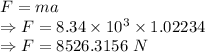
Weight of the craft

`W=mg\\\Rightarrow W=8.34* 10^3* 1.6\\\Rightarrow W=13344\ N`

Thrust

`F_t=F+W\\\Rightarrow F_t=8526.3156+13344\\\Rightarrow F_t=21870.3156\ N`

The thrust needed to reduce the velocity to zero at the instant when the craft touches the lunar surface is 21870.3156 N