Question

A survey found that 65% of callers complain about the service they receive from a call center. State the assumptions and determine the probability of each event described below.1) The next three consecutive callers complain about the service.2) The next two callers complain but not the third.3) Two out of the next three calls produce a complaint.4) None of the next 10 calls produces a complai

Answer 1

Explanation:

Given that a survey found that 65% of callers complain about the service they receive from a call center.

If x is the no of callers each person is independent of the other with equal probability 0.65

1) The next three consecutive callers complain about the service

Prob =
`=0.65^3\\=0.2746`

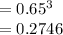
2) Next two callers complain and not thired

Prob =
`(0.65^2)(0.35)\\=0.1479`

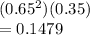
3) Two out of 3 complain

Here any two can complain so we multiply prob of 2 by 3C2

Prob =
`0.1479*3\\=0.4437`

4) Out of 10 no complaint

We can say prob

=
`(1-0.65)^(10) \\\\=2.759(10^(-5))`