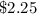(a) System of equations:
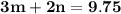 and
and
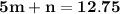
(b) One soda costs
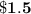
(c) One taco costs
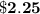
SOLUTION:
Given, Mrs. Galicia bought 3 tacos and 2 sodas and paid
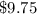 .
.
The next day she went back and bought 5 tacos and 1 soda and paid
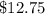 .
.
Let the cost of 1 tacos be m, and cost of 1 soda be n.
Now, We have to find
(a) System of equations:
3 tacos and 2 sodas cost
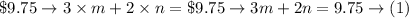
5 tacos and 1 soda cost
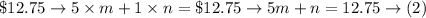
System of equations are
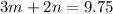
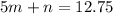
Now,
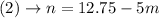
So, substitute n in (1)
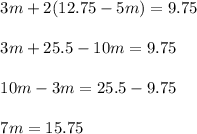
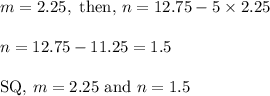
(b) cost of one soda
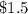
(c) cost of one taco