Question

Sponsors of a local charity decided to attract wealthy patrons to its $500-a-plate dinner by allowing each patron to buy a set of 20 tickets for the gaming tables. The chance of winning a prize for each of the 20 plays is 50–50. If you bought 20 tickets, what is the chance of winning 15 or more prizes?

Answer 1

There is a 2.06% probability of winning 15 or more prizes.

Explanation:

For each play, there are only two possible outcomes. Either you win a prize, or you do not win a prize. This means that we can solve this problem using concepts of the binomial probability distribution.

Binomial probability distribution

The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.

`P(X = x) = C_(n,x).p^(x).(1-p)^(n-x)`

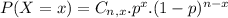
In which
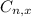`C_(n,x)` is the number of different combinatios of x objects from a set of n elements, given by the following formula.

`C_(n,x) = (n!)/(x!(n-x)!)`

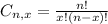
And p is the probability of X happening.

In this problem we have that:

There are 20 plays, no
`n = 20`

The chance of winning a prize for each of the 20 plays is 50–50, so
`p = 0.50`.

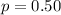If you bought 20 tickets, what is the chance of winning 15 or more prizes?

This is
`P(X \geq 15)`

`P(X \geq 15) = P(X = 15) + P(X = 16) + P(X = 17) + P(X = 18) + P(X = 19) + P(X = 20)`

`P(X = 15) = C_(20,15).(0.50)^(15).(0.50)^(5) = 0.0148`

`P(X = 16) = C_(20,16).(0.50)^(16).(0.50)^(4) = 0.0046`

`P(X = 17) = C_(20,17).(0.50)^(17).(0.50)^(3) = 0.001`

`P(X = 18) = C_(20,18).(0.50)^(18).(0.50)^(2) = 0.0002`

`P(X = 19) = C_(20,19).(0.50)^(19).(0.50)^(1) = 0.00002`

`P(X = 20) = C_(20,20).(0.50)^(20).(0.50)^(0) = 0.00000009`

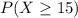
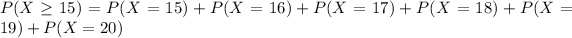
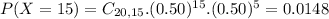
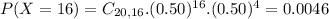
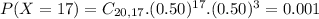
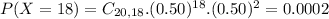
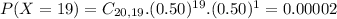
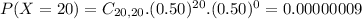
So

`P(X \geq 15) = P(X = 15) + P(X = 16) + P(X = 17) + P(X = 18) + P(X = 19) + P(X = 20) = 0.0148 + 0.0046 + 0.001 + 0.0002 + 0.00002 + 0.00000009 = 0.0206`

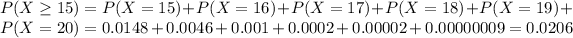
There is a 2.06% probability of winning 15 or more prizes.