Question

Which of the following equations defines a line that is parallel to the line y=-4/3x-4 and passes through the point (3,-1)

Answer 1

d

Explanation:

given that your slop is -4/3, plug the (x,y) point in to the equation of y = -4x/3 +b

-1 = -4 + b , b = 3

y = -4x/3 + 3

Answer 2

D.

Explanation:

The given line is

`y=-(4)/(3)x-4`

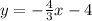
Now, if the new line is parallel to the given one, that means it must have the same slope.

`m=-(4)/(3)`

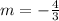
We also know that the new line passes through point (3,-1).

Then, we use the point-slope formula to find the equation of the new line:

`y-y_(1) =m(x-x_(1) )\\y-(-1)=-(4)/(3)(x-3)\\ y=-(4)/(3)x+4-1\\ y=-(4)/(3)x+3`

Therefore, the line that passes through (3,-1) and it's parallel to the given line is:
`y=-(4)/(3)x+3`.

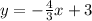The image attached show these parallel lines.