Question

Consider a small child sliding down two differently shaped slides that have the same coefficient of kinetic friction. How must the second slide be shaped to provide the same change in gravitational potential energy of the child-Earth system but more change in kinetic energy for the child while they slide down

Answer 1

Having a bigger angle above the horizontal

Step-by-step explanation:

Applying the energy conservation theorem:

`K_1+U_1+W_(ext)=K_2+U_2`

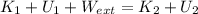
The kinetic energy is reduced because of the work done by the friction force.

The friction force is given by:

`F_f=F_N*\µ`

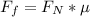
so the friction force depends on the Normal force, because the slide has an angle the normal force is given by:

`F_N=m.g*cos(\theta)`

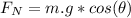
So when the angle of the slide is bigger, the friction force decreases, for example:

for 45 degrees:

`F_N=m.g*cos(45)\\F_N=0.70(m.g)\\`

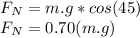
for 75 degrees:

`F_N=m.g*cos(75)\\F_N=0.26(m.g)\\`

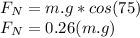
as you can see if the angle is bigger above the horizontal, the friction force is reduced and so the work done by that force. We didn't have to change the height of the slide, so the potential gravitational energy remains the same.