Question

Write in slope-intercept form an equation of the line that passes through the points (−4, 2) and (6,−3).

Answer 1

y = -0.5x

Explanation:

`\bold{slope\, (m)=(change\ in\ Y)/(change\ in\ X)=(y_2-y_1)/(x_2-x_1)}`

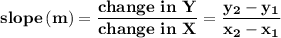
(−4, 2) ⇒ x₁ = −4, y₁ = 2

(6,−3) ⇒ x₂ = 6, y₂ = −3

So the slope:

`\bold{m=(-3-2)/(6+4)=(-5)/(10)=-0.5}`

The point-slope form of the equation of the line passing through point (x₀,y₀) and with slope m is: y - y₀ = m(x - x₀)

m = -0.5

(−4, 2) ⇒ x₀ = −4, y₀ = 2

Therefore:

y - 2 = -0.5(x - (-4))

y - 2 = -0.5x - 2 {add 2 to both sides}

y = -0.5x ← the slope-intercept form of the equation (b=0)