Answer:
B.
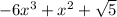
Explanation:
For an algebraic expression to be a polynomial it must have:
1)Variable
2)Constants
3) Exponents of variables which has to be whole number.
These can be combined by math operations except by division of variable
A.
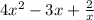
Not a polynomial the last term is a division by variable. This disqualifies it to be a polynomial.
B.
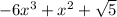
It is a polynomial as it has variables with positive exponents and a constant term.
C.
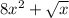
Not a polynomial the last term[
 ] has a variable with exponent which is not a whole number. This disqualifies it to be a polynomial.
] has a variable with exponent which is not a whole number. This disqualifies it to be a polynomial.
D.

Not a polynomial the last term is a division by variable. This disqualifies it to be a polynomial.