Question

a man drags a 8.10 kg bag of mulch at a constant speed, applying a 29.5 N at 38°. what is the coefficient of friction?​

Answer 1

The coefficient of friction is 0.38.

Step-by-step explanation:

The free body diagram is drawn below.

Let
`f` be frictional force acting in the backward direction as shown. Let the coefficient of friction be
`\mu`. Let
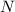`N` be the normal reaction force acting on the bag.

Given:

Mass of the bag is,
`m=8.10\textrm{ kg}`

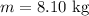
Force acting at
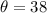`\theta = 38`° is
`F= 29.5\textrm{ N}`

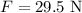
Acceleration due to gravity is,
`g=9.8\textrm{ }m/s^(2)`

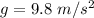
The force F can be resolved into its components as
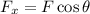`F_(x)=F \cos \theta` and
`F_(y)=F \sin \theta`

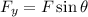
Therefore,

`F_(x)=29.5\cos(38)=23.25\textrm{ N}\\F_(y)=29.5\sin(38)=18.16\textrm{ N}`

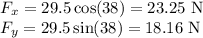
Now, as there is no acceleration in vertical direction, therefore,

Sum of upward forces = Sum of downward forces

`N+F_(y)=mg\\N=mg-F_(y)=8.10* 9.8-18.16\\N=79.38-18.16=61.22\textrm{ N}`

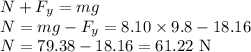
Now, as the bag is moving at a constant speed, so acceleration in the horizontal direction is also zero as acceleration is the rate of change of velocity.

Therefore, backward force = forward force.

`f=F_(x)\\f=23.25\textrm{ N}`

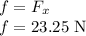
Now, frictional force is given as:

`f=\mu N\\\mu = (f)/(N)=(23.25)/(61.22)=0.38`

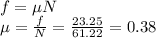
Therefore, the coefficient of friction is 0.38.