Question

The hypotenuse of right triangle is 52 centimeters long. The difference between the other two sides is 28 centimeters.

Find the missing sides. Use exact values.

Answer 1

The length of the perpendicular = 20 meters

The length of the base = 48 meters

Explanation:

The hypotenuse of the triangle = 52 meters

Let the Length of the perpendicular is = k meters

So, the length of the base = ( k + 28) m

Now, by PYTHAGORAS THEOREM , in a right angled triangle:

`(BASE)^(2)   + (PERPENDICULAR)^(2)  =  (HYPOTENUSE)^(2)`

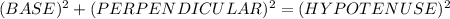
⇒ Here,
`(k)^(2) + (k +28) ^(2)  = (52)^(2)`

Also, by Algebraic Identity:

`(a+b) ^(2)  = a^(2) + b ^(2) + 2ab\\ \implies (k+28) ^(2)  = k^(2) + (28) ^(2) + 2(28)(k)\\`

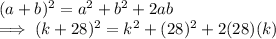
So, the equation becomes:

`(k)^(2) +k^(2) + (28) ^(2) + 2(28)(k)  = (52)^(2)`

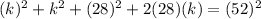
or,
`2k^(2)  + 784+ 56k = 2704\\\implies k^(2) + 28k - 960 = 0`

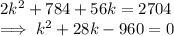
or,
`k^(2)  + 48k -20 k - 960 = 0`

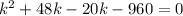
Solving the equation:

⇒ (k+48)(k-20) = 0 , or (k+48) = 0 , or (k-20) = 0

or, either k = -48 , or k = 20

As k is the length of the side, so k ≠ - 48, k = 20

Hence, the length of the perpendicular = k = 20 meters

and the length of the base is k + 28 = 48 meters