Question

Eighth grade

> T3 Ara and perimeter, word problems 6MY
A rectangular sheet of metal has an area of 247 square inches. Its perimeter is 64 inches. What are
the dimensions of the sheet?​

Answer 1

The length and width of the rectangle are 19 inches and 13 inches respectively.

Solution:

Given that , A rectangular sheet of metal has an area of 247 square inches. Its perimeter is 64 inches.

Let the length of rectangle be a and width of rectangle be b.

The area of rectangle is given as:

`area = length * width`

`\text { Then, area } \rightarrow a b=247`

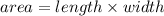
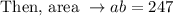
The perimeter of rectangle is given as:

Perimeter = 2(length + width)

Then 2(a + b) = 64

a + b = 32 ⇒ (1)

`\begin{array}{l}{\text { Now we know that, }(a-b)^(2)=(a+b)^(2)-4 a b} \\\\ {\text { Then, }(a-b)^(2)=32^(2)-4 * 247} \\\\ {(a-b)^(2)=1024-988} \\\\ {a-b=√(36)}\end{array}`

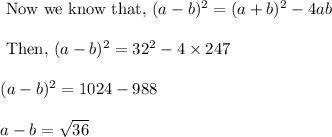
a – b = 6 ---- (2)

Now solve (1) and (2)

2a = 38

a = 19

Then, 19 + b = 32

b = 32 – 19 = 13

hence, the length and width of the rectangle are 19 inches and 13 inches respectively.