Answer:
Hence The other five trigonometric function as :
CosФ =

TanФ =
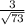
SecФ =
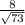
CotФ =
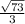
Explanation:
Given as :
sin Ф =
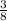
∵ sin Ф =
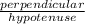
So,
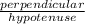 =
=
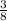
Then from Pythagorean theorem
Hypotenuse² = Perpendicular² + Base²
So, Base² = Hypotenuse² - Perpendicular²
Or, Base² = 8² + 3² = 64 + 9 = 73
∴ Base = √73
So, CosФ =
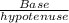
CosФ =

TanФ =
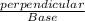
TanФ =
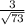
SecФ =
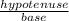
SecФ =
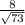
CotФ =
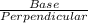
CotФ =
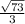
CosecФ =
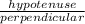
CosecФ =
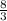
Hence The other five trigonometric function as :
CosФ =

TanФ =
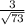
SecФ =
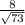
CotФ =
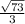
CosecФ =
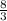 Answer
Answer