Question

Let P be the centrold of ASTU, and let SW be a medlan of ASTU. If SW = 3, find SP and PW.

The median SP is
The median PW is
ITS URGENT

Answer 1

SP = 2 and PW = 1.

Explanation:

We know that the centroid divides the medians in a 2 : 1 ratio.

Now, in Δ STU, P is the centroid and SW is a median.

So, point P will lie on median SW and will divide the median SW in a 2 : 1 ratio i.e. SP : PW = 2 : 1.

If the length of the median SW is 3 then SP =
`(3 * 2)/((1 + 2))  =2`

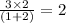
And similarly PW =
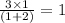`(3 * 1)/((1 + 2))  =1` (Answer)