Question

The mean checkout time in the express lane of a large grocery store is 2.7 minutes, and the standard deviation is 0.6 minutes. The distribution of checkout times is non-normal (for one thing, it can be a lot longer than 2.7 minutes, but it can only be so short).

(a) What is the probability that a randomly-selected customer will take less than 3 minutes? 0.3085 0.6915 It cannot be determined from the information given.
(b) What is the probability that the average time of two randomly-selected customers will take less than 3 minutes? 0.7611 0.2389 It cannot be determined from the information given. a
(c) The probability that the average time of 64 randomly-selected customers will take less than 2.8 minutes is.
(d) The probability that the average time of 81 randomly-selected customers will take less than 2.8 minutes is.
(e) The probability that the average time of 225 randomly-selected customers will take less than 2.8 minutes is.
(f) The probability that the average time of 400 randomly-selected customers will take less than 2.8 minutes is.

Answer 1

a) There is a 69.155 probability that a randomly-selected customer will take less than 3 minutes.

b) There is a 76.11% probability that the average time of two randomly-selected customers will take less than 3 minutes.

c) There is a 90.82% probability that the average time of 64 randomly-selected customers will take less than 2.8 minute.

d) There is a 93.19% probability that the average time of 81 randomly-selected customers will take less than 2.8 minutes.

e) There is a 99.38% probability that the average time of 225 randomly-selected customers will take less than 2.8 minutes.

f) There is a 99.95% probability that the average time of 400 randomly-selected customers will take less than 2.8 minutes.

Explanation:

Problems of normally distributed samples can be solved using the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the zscore of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.

In this problem, we have that:

The mean checkout time in the express lane of a large grocery store is 2.7 minutes, and the standard deviation is 0.6 minutes. This means that
`\mu = 2.7, \sigma = 0.6`.

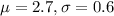(a) What is the probability that a randomly-selected customer will take less than 3 minutes?

This is the pvalue of Z when
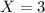`X = 3`. So:

`Z = (X - \mu)/(\sigma)`

`Z = (3 - 2.7)/(0.6)`

`Z = 0.5`

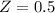
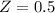`Z = 0.5` has a pvalue of 0.6915.

There is a 69.155 probability that a randomly-selected customer will take less than 3 minutes.

(b) What is the probability that the average time of two randomly-selected customers will take less than 3 minutes?

We are now working with the average of a sample, so we must use
`s` instead of
`\sigma` in the formula of Z.

`s = (\sigma)/(√(2)) = (0.6)/(√(2)) = 0.4243`

`Z = (X - \mu)/(s)`

`Z = (3 - 2.7)/(0.4243)`

`Z = 0.71`

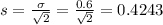
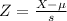
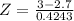
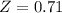
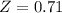`Z = 0.71` has a pvalue f 0.7611.

There is a 76.11% probability that the average time of two randomly-selected customers will take less than 3 minutes.

(c) The probability that the average time of 64 randomly-selected customers will take less than 2.8 minutes is

This is the pvalue of Z when
`X = 2.8`

`s = (\sigma)/(√(64)) = (0.6)/(8) = 0.075`

`Z = (X - \mu)/(s)`

`Z = (2.8 - 2.7)/(0.075)`

`Z = 1.33`

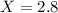
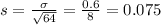
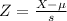
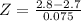
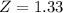
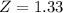`Z = 1.33` has a pvalue of 0.9082.

There is a 90.82% probability that the average time of 64 randomly-selected customers will take less than 2.8 minute.

(d) The probability that the average time of 81 randomly-selected customers will take less than 2.8 minutes is.

`s = (\sigma)/(√(81)) = (0.6)/(9) = 0.067`

`Z = (X - \mu)/(s)`

`Z = (2.8 - 2.7)/(0.067)`

`Z = 1.49`

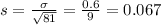
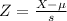
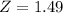
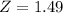`Z = 1.49` has a pvalue of 0.9319.

There is a 93.19% probability that the average time of 81 randomly-selected customers will take less than 2.8 minutes.

(e) The probability that the average time of 225 randomly-selected customers will take less than 2.8 minutes is.

`s = (\sigma)/(√(225)) = (0.6)/(15)= 0.04`

`Z = (X - \mu)/(s)`

`Z = (2.8 - 2.7)/(0.04)`

`Z = 2.50`

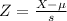
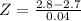
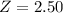
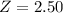`Z = 2.50` has a pvalue of 0.9938.

There is a 99.38% probability that the average time of 225 randomly-selected customers will take less than 2.8 minutes.

(f) The probability that the average time of 400 randomly-selected customers will take less than 2.8 minutes is.

`s = (\sigma)/(√(400)) = (0.6)/(20)= 0.03`

`Z = (X - \mu)/(s)`

`Z = (2.8 - 2.7)/(0.03)`

`Z = 3.30`

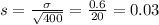
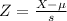
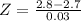
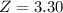
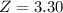`Z = 3.30` has a pvalue of 0.9995.

There is a 99.95% probability that the average time of 400 randomly-selected customers will take less than 2.8 minutes.