Question

A new surgical procedure is said to be successful 60% of the time. Suppose the operation is performed five times and the results are assumed to be independent of one another. What are the probabilities of these events? (Round your answers to three decimal places.) (a) all five operations are successful (b) exactly three are successful (c) less than two are successful

Answer 1

0.07776, 0.3456,0.08704

Explanation:

Given that a new surgical procedure is said to be successful 60% of the time

Let X be the no of successes

Then X has only two outcomes and each trial is independent of the other

Hence X is binomial with p = prob of success in a single trial = 0.60

n = Number of operations performed = 5

Prob that

a) all five operations are successful =

`P(X=5)\\= (0.6)^5\\=0.07776`

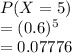
(b) exactly three are successful

=
`P(X=3) = 5C3 (0.6)^3(0.4)^2\\= 0.3456`

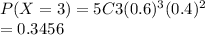
(c) less than two are successful

`==P(X<2)\\=P(x=0)+P(x=1)\\= (0.4)^5 + 5(0.6)(0.4)^4\\= 0.0256(0.4+3)\\=0.08704`