Answer:
a) Margin of error = 166.311
b) sample size ≥ 62
Explanation:
Given:
Average cost = $1599
Sample size = 50 persons
Standard deviation = $600
Confidence level is 95%
a) Margin of error =
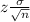
Now for confidence level of 95% z-value = 1.96
Thus,
Margin of error =
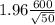
or
Margin of error = 166.311
b) For Margin of error ≤ 150
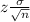 ≤ 150
≤ 150
or
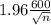 ≤ 150
≤ 150
or
 ≤ √n
≤ √n
or
√n ≥ 7.84
or
n ≥ 61.4656
Therefore,
sample size ≥ 62