Answer:

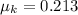
Step-by-step explanation:
It is given that,
Mass of the crate, m = 24 kg
Force acting on the crate when it is at rest,
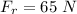
When the crate is in motion, force acting on the crate,
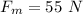
To find,
The coefficient of static friction between crate and floor
Solution,
When the crate is at rest, the force acting on the crate is given by :
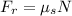
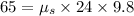

When the crate is in motion, the force acting on the crate is given by :
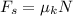
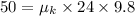
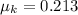
Hence, this is the required solution.