Answer :
(1) The number of grams needed of each fuel
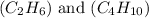 are 19.23 g and 20.41 g respectively.
are 19.23 g and 20.41 g respectively.
(2) The number of moles of each fuel
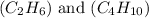 are 0.641 moles and 0.352 moles respectively.
are 0.641 moles and 0.352 moles respectively.
(3) The balanced chemical equation for the combustion of the fuels.
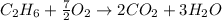

(4) The number of moles of
 produced by burning each fuel is 1.28 mole and 1.41 mole respectively.
produced by burning each fuel is 1.28 mole and 1.41 mole respectively.
The fuel that emitting least amount of
 is
is

Explanation :
Part 1 :
First we have to calculate the number of grams needed of each fuel
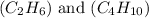 .
.
As, 52 kJ energy required amount of
 = 1 g
= 1 g
So, 1000 kJ energy required amount of
 =
=
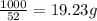
and,
As, 49 kJ energy required amount of
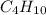 = 1 g
= 1 g
So, 1000 kJ energy required amount of
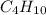 =
=
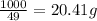
Part 2 :
Now we have to calculate the number of moles of each fuel
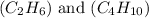 .
.
Molar mass of
 = 30 g/mole
= 30 g/mole
Molar mass of
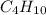 = 58 g/mole
= 58 g/mole

and,

Part 3 :
Now we have to write down the balanced chemical equation for the combustion of the fuels.
The balanced chemical reaction for combustion of
 is:
is:
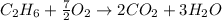
and,
The balanced chemical reaction for combustion of
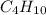 is:
is:

Part 4 :
Now we have to calculate the number of moles of
 produced by burning each fuel to produce 1000 kJ.
produced by burning each fuel to produce 1000 kJ.
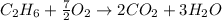
From this we conclude that,
As, 1 mole of
 react to produce 2 moles of
react to produce 2 moles of

As, 0.641 mole of
 react to produce
react to produce
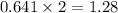 moles of
moles of

and,

From this we conclude that,
As, 1 mole of
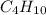 react to produce 4 moles of
react to produce 4 moles of

As, 0.352 mole of
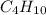 react to produce
react to produce
 moles of
moles of

So, the fuel that emitting least amount of
 is
is
